I limiti della schematizzazione "punto materiale". Forze esterne e forze interne
Febbraio 18th, 2021 | by Marcello Colozzo |
La modellizzazione di un sistema meccanico attraverso un punto materiale è utile nei seguenti casi:
- le dimensioni lineari del sistema sono trascurabili rispetto alle traiettorie seguite;
- le caratteristiche del moto sono indipendenti dalle predette dimensioni (anche se queste ultime non sono trascurabili), per cui possiamo ritenere concentrata tutta la massa del sistema in un punto.
Esistono peraltro sistemi meccanici per i quali non sono verificati i casi 1 e 2. Ad esempio, consideriamo una sfera S che compie un moto di rotazione attorno a un suo asse. Tale moto ha senso solo per un corpo esteso, per cui non è possibile schematizzare S attraverso un punto materiale. Più precisamente, la riduzione di S a un punto (ad esempio, il suo centro) porterebbe alla conclusione assurda secondo cui S è in quiete.
In molti casi, tuttavia, è possibile schematizzare il sistema meccanico in istudio, in un insieme di punti materiali. Si pensi, ad esempio, all'esplosione di un proiettile. Tale procerso può essere interpretato come la frammentazione di un corpo esteso in più parti, ciascuna della quale è modellizzabile attraverso un punto materiale. Tutto ciò ci consente di estendere il paradigma sviluppato in precedenza, ai cosiddetti sistemi di punti materiali
Ciò premesso, sussiste la seguente definizione:
Definizione
Assegnato un sistema di punti materiali, si dice configurazione del sistema, il numero di coordinate necessarie per determinare univocamente la posizione dei suoi punti. Una locuzione alternativa è numero di gradi di libertà del sistema.
Ad esempio, per un sistema costituito da n punti materiali che possono occupare una qualunque posizione nello spazio ordinario, il numero di gradi di libertà è 3n.
Lo studio del moto del sistema si riconduce a quello del moto di n punti materiali. Dobbiamo perciò distinguere le forze esterne, cioè quelle dovute a campi di forza agenti sul sistema, dalle forze interne dovute all'interazione reciproca dei singoli punti materiali.
Modeling a mechanical system through a material point is useful in the following cases:
- the linear dimensions of the system are negligible with respect to the trajectories followed;
- the characteristics of the motion are independent of the aforesaid dimensions (even if the latter are not negligible), so we can consider the whole mass of the system concentrated in one point.
However, there are mechanical systems for which cases 1 and 2 are not verified. For example, we consider a sphere S that performs a rotational motion around its axis. This motion makes sense only for an extended body, so it is not possible to schematize S through a material point. More precisely, the reduction of S to a point (for example, its center) would lead to the absurd conclusion that S is at rest.
In many cases, however, it is possible to schematize the mechanical system in the studio, in a set of material points. Think, for example, of the explosion of a bullet. This process can be interpreted as the fragmentation of a body extended into several parts, each of which can be modeled through a material point. All this allows us to extend the paradigm developed previously, to the so-called systems of material points
Definition
Given a system of material points, it is said system configuration , the number of coordinates needed to uniquely determine the position of its points. An alternative term is number of degrees of freedom of the system.
For example, for a system consisting of n material points that can occupy any position in ordinary space, the number of degrees of freedom is 3n.
The study of the motion of the system can be traced back to that of the motion of n material points. We must therefore distinguish external forces , that is, those due to force fields acting on the system, from internal forces due to the mutual interaction of the single material points.
Tags: forze esterne, foze interne, meccanica dei sistemi di punti materiali
Articoli correlati


 Congettura di Riemann
Congettura di Riemann Trasformata discreta di Fourier
Trasformata discreta di Fourier
 Trasformata di Fourier nel senso delle distribuzioni
Trasformata di Fourier nel senso delle distribuzioni Trasformata di Fourier
Trasformata di Fourier  Infinitesimi ed infiniti
Infinitesimi ed infiniti Limiti notevoli
Limiti notevoli Punti di discontinuità
Punti di discontinuità Misura di Peano Jordan
Misura di Peano Jordan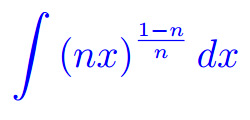 Eserciziario sugli integrali
Eserciziario sugli integrali Differenziabilità
Differenziabilità 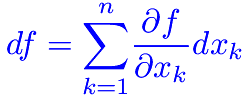 Differenziabilità (2)
Differenziabilità (2)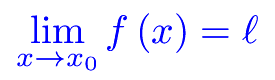 Esercizi sui limiti
Esercizi sui limiti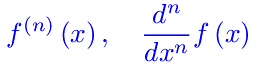 Appunti sulle derivate
Appunti sulle derivate Studio della funzione
Studio della funzione Esercizi sugli integrali indefiniti
Esercizi sugli integrali indefiniti Algebra lineare
Algebra lineare Analisi Matematica 2
Analisi Matematica 2 Analisi funzionale
Analisi funzionale Entanglement quantistico
Entanglement quantistico Spazio complesso
Spazio complesso Biliardo di Novikov
Biliardo di Novikov Intro alla Meccanica quantistica
Intro alla Meccanica quantistica Entanglement Quantistico
Entanglement Quantistico